|
C.a. Governing equations of the 1D mode
|
The potential temperature of the model atmosphere composed of ideal
gas is calculated as follows.
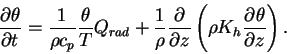 |
(C.1) |
where
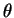 is potential temperature, is potential temperature, 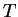 is temperature, is temperature, 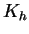 is the vertical diffusion coefficient, is the vertical diffusion coefficient, 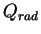 is the radiative heating rate per unit mass, and
is the radiative heating rate per unit mass, and 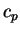 is the specific heat of constant pressure per unit mass; is the specific heat of constant pressure per unit mass;
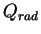 is calculated by the convergence of the radiative heat flux obtained
by the radiative transfer equation of CO2 (see
Appendix A.d.i).
Radiative transfer associated with dust is not taken into
consideration. The second term on the right-hand side represents heat
transport due to thermal convection, which is parameterized as
vertical diffusion.
is calculated by the convergence of the radiative heat flux obtained
by the radiative transfer equation of CO2 (see
Appendix A.d.i).
Radiative transfer associated with dust is not taken into
consideration. The second term on the right-hand side represents heat
transport due to thermal convection, which is parameterized as
vertical diffusion.
The vertical structure of the model atmosphere is given by the hydrostatic equation.
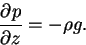 |
(C.2) |
Vertical diffusion coefficient 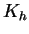 is calculated as follows (Gierasch and Goody,1968).
is calculated as follows (Gierasch and Goody,1968).
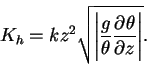 |
(C.3) |
where 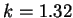 (Priestley, 1959). (Priestley, 1959).
Sensible heat flux 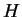 is calculated as follows (Gierasch and Goody, 1968).
is calculated as follows (Gierasch and Goody, 1968).
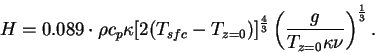 |
(C.4) |
where 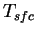 is the surface temperature, is the surface temperature, 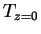 is the surface atmospheric temperature, is the surface atmospheric temperature, 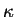 is the atmospheric thermal diffusion coefficient,
and is the atmospheric thermal diffusion coefficient,
and 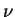 is atmospheric kinematic viscosity.
The values of
is atmospheric kinematic viscosity.
The values of
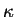 and and
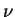 are
8×10-4 m2sec-1 and
1×10-3 m2sec-1,
respectively
(Gierasch and Goody, 1968).
Sensible heat flux are
8×10-4 m2sec-1 and
1×10-3 m2sec-1,
respectively
(Gierasch and Goody, 1968).
Sensible heat flux
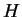 is 0 when the surface atmospheric temperature is larger than the surface temperature.
is 0 when the surface atmospheric temperature is larger than the surface temperature.
The surface temperature
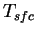 is calculated by using the thermal diffusion equation (see
Appendix A.e).
is calculated by using the thermal diffusion equation (see
Appendix A.e).
|