Development of a Cloud Convection Model for Jupiter's Atmosphere << Prev | Index| Next >>

F. Calculation of Thermodynamical Properties

Saturation vapor pressure and latent heat of H2O and NH3

Saturation vapor pressure

The expression of saturation vapor pressure pvsw is adopted from eq. (14) of Briggs and Sacket (1989) [17].

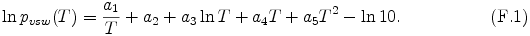
The coefficients a1 - a5 in eq. (F.1) are given by Table F.1. Note that the term -ln10 is added from the expressoin of Briggs and Sacket (1989) [17], since SI units are used instead of cgs units.

Latent Heat

The latent heat per mole Lv is represented by using Clausius-Clapeyron equation and eq. (F.1) as follows.

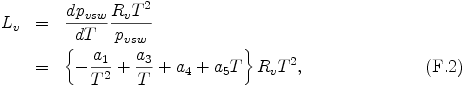
where Rv is the gas constant of each condensible species.

Table F.1: The coefficients in eqs. (F.1) and (F.2) (Briggs and Sacket, 1989 [17]).

Species a1 a a3 a4 a5
NH3(s) -4122 41.67871 -1.81630 0 0
H2O(s) -5631.1206 -8.363602 8.2312 -3.861449 × 10-2 2.77494 × 10-5

Equilibrium constant and reaction heat of NH4SH

Equilibrium constant

The equilibrium constant Kp in the NH4SH production reaction, H2S(g) + NH3(g) ↔ NH4SH(s), is adopted from eq. (19) of Briggs and Sacket (1989) [17].

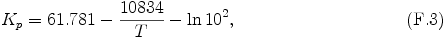
The term -ln102 has been added since SI units are used instead of cgs units.

Reaction Heat

The reaction heat per unit mol LNH4SH is represented by using the van't Hoff equation and eq. (F.3) as follows.

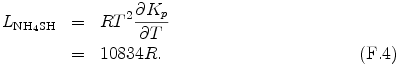
where R is universal gas constant.

Development of a Numerical Model for Jupiter's Atmosphere << Prev | Index| Next >>