5.3.2 鉛直流の生成への寄与
続いて、鉛直流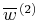 の生成要因を調べる。(23)〜(25)より、
の生成要因を調べる。(23)〜(25)より、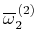 について調べれば良い。(17)の
について調べれば良い。(17)の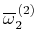 成分について書き下すと次のようになる。
成分について書き下すと次のようになる。
第2項(基本流と二次流の相互作用の効果)はゼロとなる。第1項(擾乱同士の相互作用: 運動量輸送の効果)、第3項(二次流に働くコリオリ力の効果)、第4項(浮力の効果)、第5項(粘性の効果)、右辺の総和の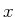 -
-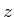 分布を図27(
分布を図27(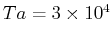 の場合は図29)に示す。このままでは分かりにくいが、実際にコリオリ力が働く
の場合は図29)に示す。このままでは分かりにくいが、実際にコリオリ力が働く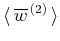 の
の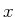 分布は図28(
分布は図28(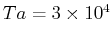 の場合は図30)のようになる((24)と非圧縮条件を用いて
の場合は図30)のようになる((24)と非圧縮条件を用いて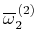 から変換)。これを見ると、第4項の浮力の効果の寄与が一番大きいのが分かる。すなわち、固有モードの二次効果によって生成された浮力偏差の水平(
から変換)。これを見ると、第4項の浮力の効果の寄与が一番大きいのが分かる。すなわち、固有モードの二次効果によって生成された浮力偏差の水平(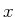 方向)勾配が、
方向)勾配が、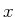 -
-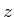 面内の循環の生成に大きく寄与する。
面内の循環の生成に大きく寄与する。
第1項 (CONTOUR INTERVAL
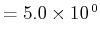
)
第3項 (CONTOUR INTERVAL
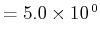
)
第4項 (CONTOUR INTERVAL
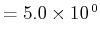
)
第5項 (CONTOUR INTERVAL
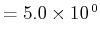
)
総和 (CONTOUR INTERVAL
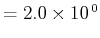
)
図27:
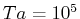 の場合の発達率最大の固有モードによる、
の場合の発達率最大の固有モードによる、 への各項の寄与。
への各項の寄与。
上から順に、第1項(擾乱同士の相互作用: 運動量輸送の効果)、第3項(二次流
に働くコリオリ力の効果)、第4項(浮力の効果)、第5項(粘性の効果)、総和
|
図28:
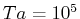 の場合の発達率最大の固有モードによる、
の場合の発達率最大の固有モードによる、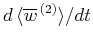 への各項の寄与。
への各項の寄与。
赤線: 第1項(擾乱同士の相互作用: 運動量輸送の効果)、緑線: 第3項(二次流に働くコリオリ力の効果)、
青線: 第4項(浮力の効果)、紫線: 第5項(粘性の効果)、水色線: 総和(図25の緑線と対応)。
|
|
第1項 (CONTOUR INTERVAL
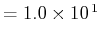
)
第3項 (CONTOUR INTERVAL
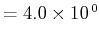
)
第4項 (CONTOUR INTERVAL
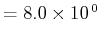
)
第5項 (CONTOUR INTERVAL
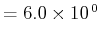
)
総和 (CONTOUR INTERVAL
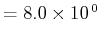
)
図29:
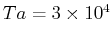 の場合の発達率最大の固有モードによる、
の場合の発達率最大の固有モードによる、 への各項の寄与。
への各項の寄与。
上から順に、第1項(擾乱同士の相互作用: 運動量輸送の効果)、第3項(二次流
に働くコリオリ力の効果)、第4項(浮力の効果)、第5項(粘性の効果)、総和
|
図30:
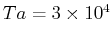 の場合の発達率最大の固有モードによる、
の場合の発達率最大の固有モードによる、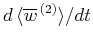 への各項の寄与。
への各項の寄与。
赤線: 第1項(擾乱同士の相互作用: 運動量輸送の効果)、緑線: 第3項(二次流に働くコリオリ力の効果)、
青線: 第4項(浮力の効果)、紫線: 第5項(粘性の効果)、水色線: 総和(図26の緑線と対応)。
|
|
SAITO Naoaki
2009-07-09
![]() の生成要因を調べる。(23)〜(25)より、
の生成要因を調べる。(23)〜(25)より、![]() について調べれば良い。(17)の
について調べれば良い。(17)の![]() 成分について書き下すと次のようになる。
成分について書き下すと次のようになる。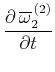
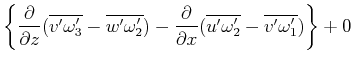
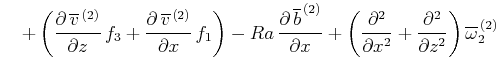
![\begin{figure}\begin{center}
\protect\includegraphics[trim=155 0 190 0,clip,scale=0.5]{img2/ksA-e-FX/f316-e-FX2-o2-3.ps}
\end{center}\end{figure}](img276.png)
![\begin{figure}\begin{center}
\protect\includegraphics[trim=155 0 190 0,clip,scale=0.5]{img2/ksA-e-FX/f316-e-FX2-o2-4.ps}
\end{center}\end{figure}](img277.png)
![\begin{figure}\begin{center}
\protect\includegraphics[trim=155 0 190 0,clip,scale=0.5]{img2/ksA-e-FX/f316-e-FX2-o2-5.ps}
\end{center}\end{figure}](img278.png)
![\begin{figure}\begin{center}
\protect\includegraphics[trim=155 0 190 0,clip,scale=0.5]{img2/ksA-e-FX/f316-e-FX2-o2-6.ps}
\end{center}\end{figure}](img279.png)
![\includegraphics[trim=0 0 5 4,clip,scale=1.28]{img2/ksA-ph15-f316-e-FX2-w2.ps}](img283.png)
![\begin{figure}\begin{center}
\protect\includegraphics[trim=155 0 190 0,clip,scale=0.5]{img2/ksA-e-FX/f173-e1-FX2-o2-3.ps}
\end{center}\end{figure}](img286.png)
![\begin{figure}\begin{center}
\protect\includegraphics[trim=155 0 190 0,clip,scale=0.5]{img2/ksA-e-FX/f173-e1-FX2-o2-4.ps}
\end{center}\end{figure}](img288.png)
![\begin{figure}\begin{center}
\protect\includegraphics[trim=155 0 190 0,clip,scale=0.5]{img2/ksA-e-FX/f173-e1-FX2-o2-5.ps}
\end{center}\end{figure}](img290.png)
![\begin{figure}\begin{center}
\protect\includegraphics[trim=155 0 190 0,clip,scale=0.5]{img2/ksA-e-FX/f173-e1-FX2-o2-6.ps}
\end{center}\end{figure}](img292.png)
![\includegraphics[trim=0 0 5 4,clip,scale=1.28]{img2/ksA-ph15-f173-e1-FX2-w2.ps}](img293.png)